Lecture 상태변수 피드백 제어기 예제
Lecture
• Views 768
• Comments 0
• Created at 2 years ago
• Last Updated at 1 year ago
- 피드백 제어기
이 시스템은 단위계단응답 37.5%의 오버슈트와 4.3초의 정정시간을 가진다. 이 시스템이 오버슈트가 9.48%, 정정시간 1초이하되도록 제어기를 설계하여라.
답)
- 플랜트의 오버슈트와 정정시간을 확인 - 오버슈트 37.5%, 정정시간 4.3초
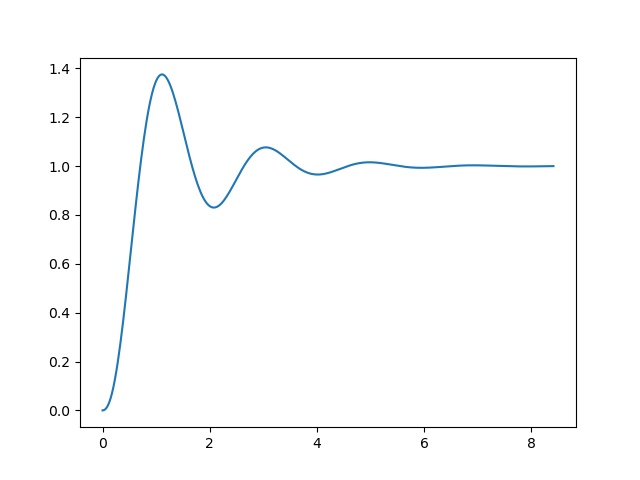
- 오버슈트 9.48%와 정정시간 1초가 되는 시스템이 되는 을 구한다.
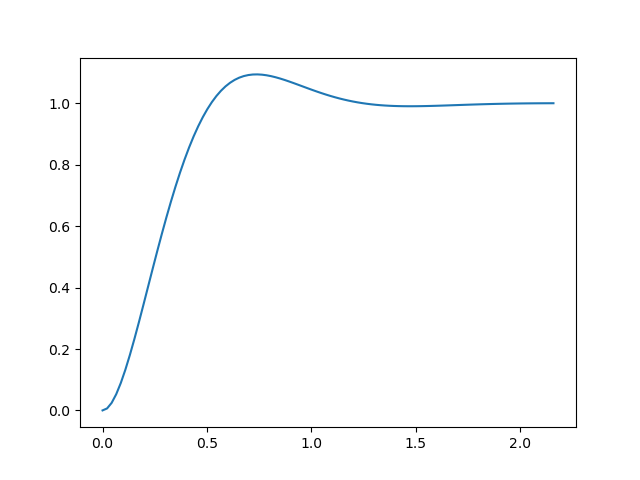
위 시스템의 극점 :
에 하나의 극점을 추가 -> 플랜트가 3차이기 때문임
액커만 공식을 이용하여 K값을 구함
새로운 시스템은
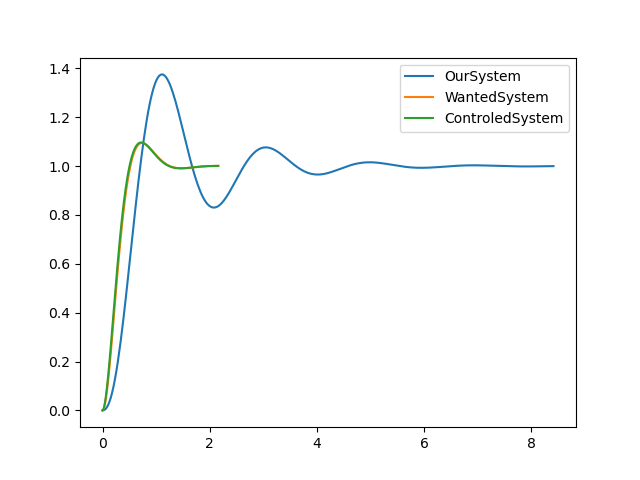
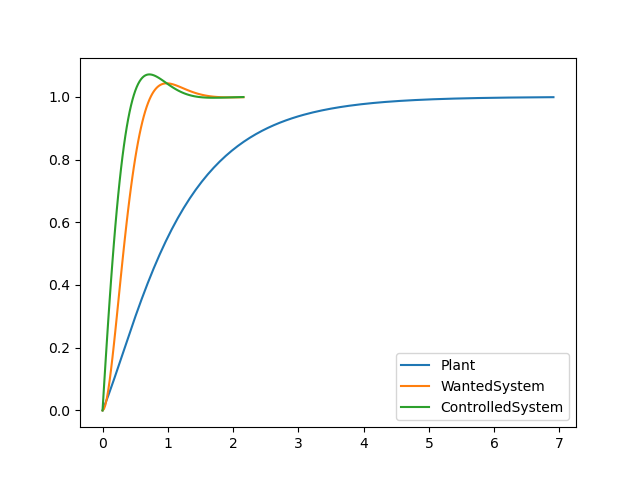
예제) 다음 시스템의 백분율 오버슈트 4.3%, 정정시간 1초가 되도록 제어기를 설계하여라.
답)
예제 9.5)
가 되도록 상태부귀환 이득벡터를 구하라.
답)
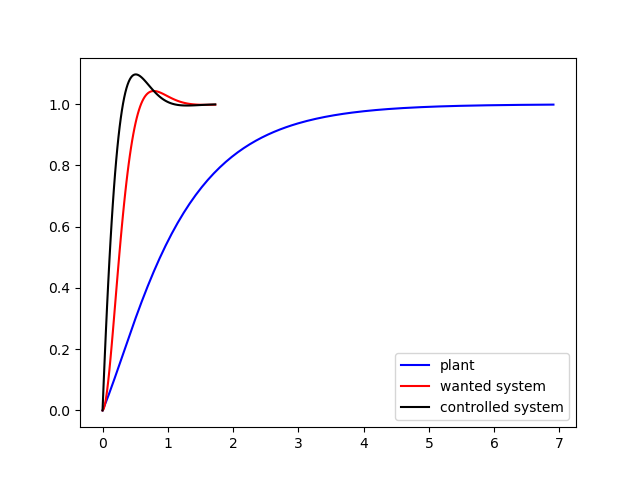
예제) 다음 시스템의 오버슈트가 10%, 정정시간 0.8초이하가 되도록 제어기를 설계하여라.
previous article
next article
Login to write a comment.